Efficient optimization design method of helicopter rotor airfoil
-
摘要:
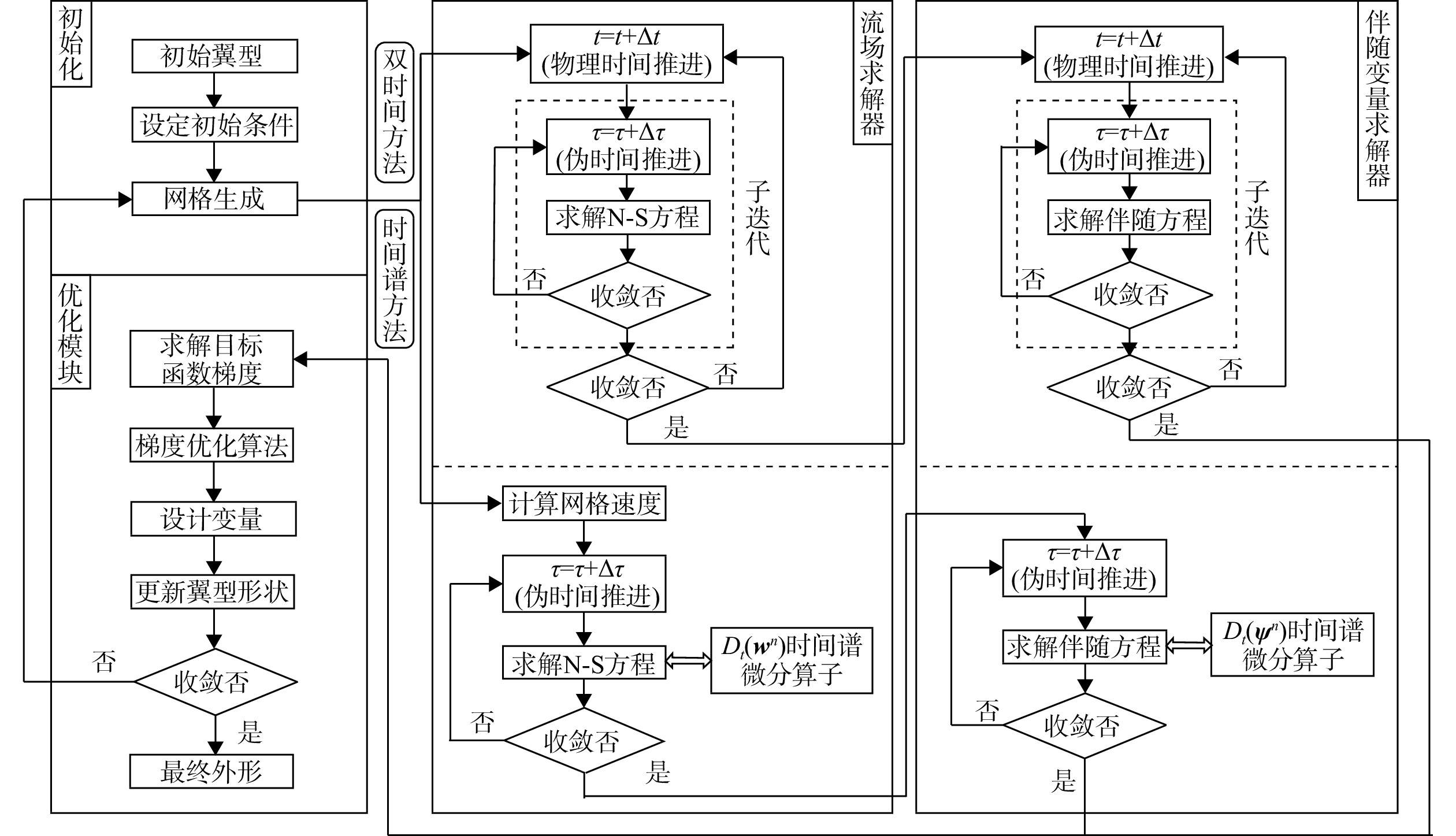
基于传统双时间方法的共轭梯度旋翼翼型优化设计方法效率低下，难以满足工程实际多点多工况的优化需求，针对直升机旋翼翼型非定常多点多目标优化设计问题，耦合高效的时间谱方法和多重网格方法，发展了一种适用于直升机旋翼翼型悬停、前飞和机动等多种运动状态的多点多目标优化设计方法，其中，Navier-Stokes方程和共轭方程的求解均采用时间谱方法进行物理时间项的离散，同时还采用几何多重网格加速收敛，以提翼型优化计算效率。算例选取典型旋翼翼型NACA0012与OA209分别开展悬停、前飞和机动等状态的定常优化与非定常优化。结果表明，该静态与动态气动外形优化设计方法具有较高精度，能够实现直升机旋翼的悬停、机动和前飞等复杂运动状态下的翼型多点多目标优化设计；相比于传统的双时间共轭梯度优化设计方法，时间谱共轭梯度优化设计方法能够提高翼型优化计算效率5倍以上。
Abstract:The adjoint-based design optimization method of rotor airfoil is inefficient in combination with a dual time stepping method, making it difficult to meet the optimization requirements of multi-point and multi-objective optimization in engineering. Considering the problem of unsteady optimization design of rotor airfoil, coupled with efficient time spectral method and multigrid method, a multi-point and multi-objective optimization design method suitable for multiple motion states of helicopter, such as hovering, forward flight and maneuvering, was developed. The Navier-Stokes equation and adjoint equation were solved by using the time spectral method to discretize the physical time term. In addition, the multigrid method was used to improve the optimization efficiency. The rotor airfoil NACA0012 and OA209 were selected to carry out multi-point, multi-objective steady and unsteady optimizations. The results showed that the static and dynamic aerodynamic shape optimization design methods had high accuracy, and can realize the multi-point and multi-objective optimization design of rotor airfoils under complex motion states; compared with dual time stepping and adjoint-based design optimization method, the time spectral and adjoint-based design optimization method can improve the calculation efficiency of airfoil optimization by more than 5 times.
-
Key words:
- rotor /
- airfoil /
- optimization design /
- adjoint method /
- time spectral method
-
图 2 非定常气动力计算值与实验值对比
Figure 2. Comparison of calculated data of unsteady aerodynamic force with test data
图 4 定常状态下优化前后翼型的几何外形对比图
Figure 4. Comparison of airfoil before and after optimization under the steady condition
图 5 定常状态下优化前后翼型气动特性对比（
$ Ma = 0.6 $ ）Figure 5. Comparison of aerodynamic characteristics of airfoil before and after optimization under the steady condition （
$ Ma = 0.6 $ ）
图 6 优化前后翼型极曲线对比图（
$ Ma = 0.6 $ ）Figure 6. Drag polar comparison of airfoil before and afteroptimization （
$ Ma = 0.6 $ ）
图 7 定常状态下优化前后翼型表面压力系数分布对比图（
$ Ma = 0.4 $ ）Figure 7. Comparison of pressure coefficient distribution of airfoil before and after optimization under the steady condition （
$ Ma = 0.4 $ ）
图 8 定常状态下优化前后翼型气动特性对比图（
$ Ma = 0.4 $ ）Figure 8. Comparison of aerodynamic characteristics of airfoil before and after optimization under the steady consition （
$ Ma = 0.4 $ ）
图 9 非定常状态下优化前后翼型表面压力系数分布对比图（
$ Ma = 0.4 $ ）Figure 9. Comparison of pressure coefficient distribution of airfoil before and after optimization under the unsteady consition （
$ Ma = 0.4 $ ）
图 10 非定常状态下优化前后翼型的几何外形对比图（算例1）
Figure 10. Comparison of airfoil before and after optimization under the unsteady consition （case 1）
图 11 非定常状态下优化前后翼型气动特性对比图（算例1）
Figure 11. Comparison of aerodynamic characteristics of airfoil before and after optimization under the unsteady consition （case 1）
图 12 非定常状态下优化前后翼型的几何外形对比图（算例2）
Figure 12. Comparison of airfoil before and after optimization under the unsteady condition（case 2）
表 1 振荡翼型计算工况
Table 1. Computation condition for oscillating airfoils
Ma $ {\alpha _{\mathrm{m}}} $/（°） $ {\alpha _0}$/（°） kf Re/106 0.6 2.89 2.41 0.0808 4.8 
下载: 导出CSV
表 2 设计状态、目标函数及约束条件
Table 2. Design states, objective functions and constraint conditions
状态编号 设计状态 目标函数 约束条件 状态1 $ Ma = 0.6 $ $ \dfrac{{{C_{\mathrm{l}}}}}{{{C_{\mathrm{d}}}}} < {\left[ {\dfrac{{{C_{\mathrm{l}}}}}{{{C_{\mathrm{d}}}}}} \right]_0} $ $ {C_{\mathrm{l}}} > {C_{{\mathrm{l}}0}} $ $ {C_{\mathrm{l}}} = 0.6 $ 状态2 $ Ma = 0.4 $ $ {C_{{\mathrm{l}},\max }} > {C_{{\mathrm{l}},\max 0}} $ $ {C_{\mathrm{d}}} < {C_{{\mathrm{d}}0}} $ $ \alpha = 11^\circ $ $ \left| {{C_{\mathrm{m}}}} \right| < \left| {{C_{{\mathrm{m}}0}}} \right| $ 注：表中下标0表示初始翼型的气动性能，下标max表示取最大值。
下载: 导出CSV
表 3 优化翼型与初始翼型气动特性计算值
Table 3. Calculated data of aerodynamic characteristic of optimized airfoil and initial airfoil
状态编号
（参数）初始翼型 优化翼型 优化
百分比/%状态1（$ {{{C_{\mathrm{l}}}} /{{C_{\mathrm{d}}}}} $） 48.09 49.47 2.87 状态2（$ {C_{\mathrm{l}}} $） 1.075 1.102 2.48
下载: 导出CSV
表 4 非定常优化设计工况
Table 4. Unsteady optimum design condition
算例 翼型 Ma $ {\alpha _{\mathrm{m}}} $/（°） $ {\alpha _0} $/（°） kf 1 NACA0012 0.6 2.89 2.41 0.0808 2 OA209 0.4 8 6 0.07
下载: 导出CSV
表 5 优化翼型与初始翼型时均气动特性计算值（算例1）
Table 5. Calculated data of time averaged aerodynamic forces of optimized airfoil and initial airfoil （case 1）
时均气动力 初始翼型 优化翼型1 优化翼型2 $ \overline {{C_{\mathrm{l}}}} $ 0.378 0.380 0.386 $ \overline {{C_{\text{d}}}} $ 0.0369 0.0363 0.0361 $ \left| {\overline {{C_{\mathrm{m}}}} } \right| $ 0.00851 0.00353 0.00781
下载: 导出CSV
表 6 优化翼型与初始翼型时均气动特性计算值（算例2）
Table 6. Calculated data of time averaged aerodynamic forces of optimized airfoil and initial airfoil （case 2）
时均气动力 初始翼型 优化翼型1 优化翼型2 $ \overline {{C_{\mathrm{l}}}} $ 0.782 0.804 0.836 $ \overline {{C_{\text{d}}}} $ 0.2013 0.1957 0.1936 $ \left| {\overline {{C_{\mathrm{m}}}} } \right| $ 0.05341 0.03264 0.04575
下载: 导出CSV
-
[1] 张卫国,孙俊峰,招启军,等. 旋翼翼型气动设计与验证方法[J]. 空气动力学学报,2021,39(6): 136-148,155. ZHANG Weiguo,SUN Junfeng,ZHAO Qijun,et al. Aerodynamic design and verification methods of rotor airfoils[J]. Acta Aerodynamica Sinica,2021,39(6): 136-148,155. (in Chinese doi: 10.7638/kqdlxxb-2021.0315ZHANG Weiguo, SUN Junfeng, ZHAO Qijun, et al. Aerodynamic design and verification methods of rotor airfoils[J]. Acta Aerodynamica Sinica, 2021, 39(6): 136-148, 155. (in Chinese) doi: 10.7638/kqdlxxb-2021.0315 [2] JAMESON A. Aerodynamic design via control theory[J]. Journal of Scientific Computing,1988,3(3): 233-260. doi: 10.1007/BF01061285 [3] ANDERSON W K,VENKATAKRISHNAN V. Aerodynamic design optimization on unstructured grids with a continuous adjoint formulation[J]. Computers & Fluids,1999,28(4/5): 443-480. [4] NADARAJAH S,JAMESON A. A comparison of the continuous and discrete adjoint approach to automatic aerodynamic optimization[R]. AIAA 2000-667,2000. [5] 杨旭东. 基于控制理论的气动优化设计技术研究[D]. 西安: 西北工业大学,2002. YANG Xudong. Research on aerodynamic optimization design technology based on control theory[D]. Xi’an: Northwestern Polytechnical University,2002. (in ChineseYANG Xudong. Research on aerodynamic optimization design technology based on control theory[D]. Xi’an: Northwestern Polytechnical University, 2002. (in Chinese) [6] 左英桃,高正红,何俊. 基于N-S方程和离散共轭方法的气动外形设计[J]. 空气动力学学报,2010,28(5): 509-512,524. ZUO Yingtao,GAO Zhenghong,HE Jun. Aerodynamic design method based on N-S equations and discrete adjoint approach[J]. Acta Aerodynamica Sinica,2010,28(5): 509-512,524. (in Chinese doi: 10.3969/j.issn.0258-1825.2010.05.005ZUO Yingtao, GAO Zhenghong, HE Jun. Aerodynamic design method based on N-S equations and discrete adjoint approach[J]. Acta Aerodynamica Sinica, 2010, 28(5): 509-512, 524. (in Chinese) doi: 10.3969/j.issn.0258-1825.2010.05.005 [7] 黄海生,杨旭东. 基于控制理论的旋翼翼型优化设计[J]. 航空计算技术,2010,40(4): 22-26. HUANG Haisheng,YANG Xudong. Optimum shape design of rotor airfoils via control theory[J]. Aeronautical Computing Technique,2010,40(4): 22-26. (in Chinese doi: 10.3969/j.issn.1671-654X.2010.04.007HUANG Haisheng, YANG Xudong. Optimum shape design of rotor airfoils via control theory[J]. Aeronautical Computing Technique, 2010, 40(4): 22-26. (in Chinese) doi: 10.3969/j.issn.1671-654X.2010.04.007 [8] 吴琪. 基于粘性伴随方法的旋翼先进气动外形优化设计分析[D]. 南京: 南京航空航天大学,2014. WU Qi. Optimal design and analysis on advanced aerodynamic shape of rotor based on A viscous adjoint method[D]. Nanjing: Nan-jing University of Aeronautics and Astronautics,2014. (in ChineseWU Qi. Optimal design and analysis on advanced aerodynamic shape of rotor based on A viscous adjoint method[D]. Nanjing: Nan-jing University of Aeronautics and Astronautics, 2014. (in Chinese) [9] MANI K,LOCKWOOD B,MAVRIPLIS D. Adjoint-based unsteady airfoil design optimization with application to dynamic stall[C]// American Helicopter Society 68th Annual Forum. FortWorth,Texas, US: American Helicopter Society International,Inc,2012: 68. [10] WANG Qing,ZHAO Qijun,WU Qi. Aerodynamic shape optimization for alleviating dynamic stall characteristics of helicopter rotor airfoil[J]. Chinese Journal of Aeronautics,2015,28(2): 346-356. doi: 10.1016/j.cja.2014.12.033 [11] 招启军,王清,赵国庆. 旋翼翼型定常-非定常特性综合优化设计新方法[J]. 南京航空航天大学学报,2014,46(3): 355-363. ZHAO Qijun,WANG Qing,ZHAO Guoqing. New optimization design method for rotor airfoil considering steady-unsteady characteristics[J]. Journal of Nanjing University of Aeronautics & Astronautics,2014,46(3): 355-363. (in Chinese doi: 10.3969/j.issn.1005-2615.2014.03.004ZHAO Qijun, WANG Qing, ZHAO Guoqing. New optimization design method for rotor airfoil considering steady-unsteady characteristics[J]. Journal of Nanjing University of Aeronautics & Astronautics, 2014, 46(3): 355-363. (in Chinese) doi: 10.3969/j.issn.1005-2615.2014.03.004 [12] 王清,招启军. 基于遗传算法的旋翼翼型综合气动优化设计[J]. 航空动力学报,2016,31(6): 1486-1495. WANG Qing,ZHAO Qijun. Synthetical optimization design of rotor airfoil by genetic algorithm[J]. Journal of Aerospace Power,2016,31(6): 1486-1495. (in ChineseWANG Qing, ZHAO Qijun. Synthetical optimization design of rotor airfoil by genetic algorithm[J]. Journal of Aerospace Power, 2016, 31(6): 1486-1495. (in Chinese) [13] HALL K C,THOMAS J P,CLARK W S. Computation of unsteady nonlinear flows in cascades using a harmonic balance technique[J]. AIAA Journal,2002,40: 879-886. doi: 10.2514/2.1754 [14] GOPINATH A,JAMESON A. Time spectral method for periodic unsteady computations over two- and three- dimensional bodies[R]. AIAA 2005-1220,2005. [15] GOPINATH A,JAMESON A. Application of the time spectral method to periodic unsteady vortex shedding[R]. AIAA 2006-449,2006. [16] 杨小权,程苏堃,杨爱明,等. 基于时间谱方法的振荡翼型和机翼非定常黏性绕流数值模拟[J]. 航空学报,2013,34(4): 787-797. YANG Xiaoquan,CHENG Sukun,YANG Aiming,et al. Time spectral method for numerical simulation of unsteady viscous flow over oscillating airfoil and wing[J]. Acta Aeronautica et Astronautica Sinica,2013,34(4): 787-797. (in ChineseYANG Xiaoquan, CHENG Sukun, YANG Aiming, et al. Time spectral method for numerical simulation of unsteady viscous flow over oscillating airfoil and wing[J]. Acta Aeronautica et Astronautica Sinica, 2013, 34(4): 787-797. (in Chinese) [17] 杨小权,杨爱明,翁培奋. 悬停旋翼无粘流场数值模拟中的多重网格方法[J]. 空气动力学学报,2009,27(5): 608-615. YANG Xiaoquan,YANG Aiming,WENG Peifen. The mutilgrid method in Euler equation computation about a helicopter rotor in hover[J]. Acta Aerodynamica Sinica,2009,27(5): 608-615. (in Chinese doi: 10.3969/j.issn.0258-1825.2009.05.019YANG Xiaoquan, YANG Aiming, WENG Peifen. The mutilgrid method in Euler equation computation about a helicopter rotor in hover[J]. Acta Aerodynamica Sinica, 2009, 27(5): 608-615. (in Chinese) doi: 10.3969/j.issn.0258-1825.2009.05.019 [18] 姬昌睿,杨小权,杨爱明,等. 用强耦合RANS方法模拟旋翼悬停流场[J]. 航空动力学报,2014,29(8): 1894-1903. JI Changrui,YANG Xiaoquan,YANG Aiming,et al. Strongly coupled RANS algorithm for simulating hovering rotor flow[J]. Journal of Aerospace Power,2014,29(8): 1894-1903. (in ChineseJI Changrui, YANG Xiaoquan, YANG Aiming, et al. Strongly coupled RANS algorithm for simulating hovering rotor flow[J]. Journal of Aerospace Power, 2014, 29(8): 1894-1903. (in Chinese) [19] PIRONNEAU O. On optimum profiles in Stokes flow[J]. Journal of Fluid Mechanics,1973,59(1): 117-128. doi: 10.1017/S002211207300145X [20] HICKS R M,HENNE P A. Wing design by numerical optimization[J]. Journal of Aircraft,1978,15(7): 407-412. doi: 10.2514/3.58379 -
点击查看大图
计量
- 文章访问数: 34
- HTML浏览量: 21
- PDF量: 5
- 被引次数: 0